Conic Sections - Online Test
Equation of circle is
Applying completing the square method
Comparing the above equation with we get center as (-3,3) and radius as .
As centre of the circlre lies on diameter , it will satisfy the equation of diameter, so on putting (-3,3) in equation of diameter we get
=>
=>
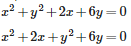
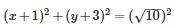
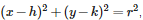 we get its center as (-1,-3) and radius (r1)=
we get its center as (-1,-3) and radius (r1)=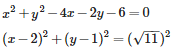
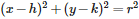 we get its center as (2,1) and radius (r2)=
we get its center as (2,1) and radius (r2)=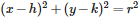 units
units and putting the values of r1, r2 and d we get length as
and putting the values of r1, r2 and d we get length as 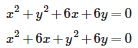
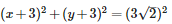
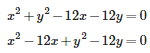
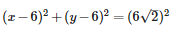
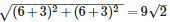 and sum of radii
and sum of radii 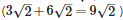 are equal.
are equal.Hence the circle touches externally.
The equation represents
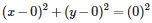 Here the center is (0,0) and radius is also 0 units.
Here the center is (0,0) and radius is also 0 units.So it is a degenerate circle as degenerate circle is a circle( a point) where radius is zero units.
The equation represents
The general equation of the circle is x2+y2-2gh-2fy+c = 0. Sice the given equations satisfies the general equation, it represents the equation of the circle.
circumcentre of a right angled triangle ABC right angled at A is as circumcentre of right angled triangle lies on the mid pont of the hypotenuse.
so mid point of BC=(,) i.e.(3,2)
(x-a)2 + (y-b)2 = c2 here (a,b) is center and c is the radius
and radius cannot be zero because if radius is zero it will become a point or degenerate circle so c0.


so center is (4,3) and radius is 4.
Distance between center and given point 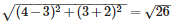 which is greater than 4.
which is greater than 4.
hence point lies outside the circle .
Since point lies outside of the circle there will be 2 tangents since two tangents can be drawn from external point to a circle.
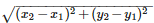
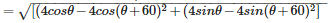 on simplifying we get,
on simplifying we get,