Number System - Online Test
Let the number be (10x + y), so when the digits of number are reversed the number becomes (10y + x).
According the question, (10y + x) – (10x + y) = 18
⇒ 9 (y – x) = 18 ⇒y – x = 2
So, the possible pairs of (x, y) are
(1, 3), (2, 4), (3, 5), (4, 6), (5,7), (6, 8) and (7,9).
But we want the number other than 13.
Thus, there are 6 possible numbers, i.e., 24, 35, 46, 57, 68, 79.
So, total number of possible numbers are 6.
Let the rectangle has x and y tiles along its length and breadth respectively.
The number of white tiles
W = 2x + 2(y – 2) = 2 (x + y – 2)
And the number of red tiles = R = xy – 2 (x + y – 2)
Given that the number of white tiles is the same as the number of red tiles
⇒ 2 (x + y – 2) = xy – 2 (x + y – 2)
⇒ 4 (x + y – 2) = xy
⇒ xy – 4x – 4y = –8
⇒ (x – 4) (y – 4) = 8 = 8 ×1 or 4 × 2
⇒m – 4 = 8 or 4 ⇒m = 12 or 8
Therefore.the number of tiles along one edge of the floor can be 12
Given, Number of adults > Number of boys > Number of girls > Number of families.
Going back from the choices, let us start with the least value given in the choices.
Since the minimum possible number of families has been asked.
In choice (c), Number of families = 2
⇒Number of girls > 3, Number of boys > 4 and Number of adults > 5
But two families together can have a maximum of 4 adults.
Therefore, the number of families is not equal to 2.
In Choice (d). Number of families = 3.
Therefore, the Number of (girls) > 4. Number of (boys) > 5 and Number of (adults) > 6
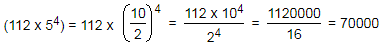
Let 232 = x. Then, (232 + 1) = (x + 1).
Let (x + 1) be completely divisible by the natural number N. Then,
(296 + 1) = [(232)3 + 1] = (x3 + 1) = (x + 1)(x2 - x + 1), which is completely divisible by N, since (x + 1) is divisible by N.
| 1397 x 1397 | = (1397)2 |
| = (1400 - 3)2 | |
| = (1400)2 + (3)2 - (2 x 1400 x 3) | |
| = 1960000 + 9 - 8400 | |
| = 1960009 - 8400 | |
| = 1951609. |